Gravitational forces and a tidal bulge
Newton's law of universal gravitation tells us that the strength of the gravitational force between two bodies is a function of their masses and the distance between them. This law also states that the direction of the gravitational attraction force is along the line joining the two bodies.
The Earth and moon are held together by gravitational attraction, which is balanced at the common centre of gravity by an equal and opposite centrifugal force. Away from the centre of gravity the strength of the gravitational force changes as the distance to the moon varies. The centrifugal force, however, remains constant everywhere on Earth. Therefore, except at the centre of gravity, the gravitational and centrifugal forces are not the same and this inequality creates a residual force. These three forces are depicted in the following diagram.
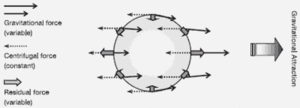
If the Earth were completely covered by a very deep ocean this residual force would act on the water and produce two bulges, one on the side facing the moon and one on the opposite side of the Earth.
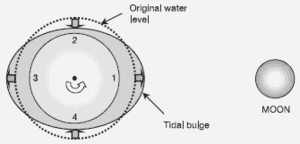
The following diagram shows the Earth and moon as viewed from above the North Pole. An observer under the water on the Earth at position 1 is beneath one of the water bulges and would be experiencing a high tide. A quarter of a revolution later, at position 2 where the original water level has been depressed, a low tide would be seen. The high tide, low tide sequence repeats as the observer moves around through points 3 and 4.
The Earth-sun system is also subject to similar gravitational and centrifugal forces but due to the sun's greater distance they have less than half the strength of the lunar-related forces. As a consequence, the solar-related residual forces and resulting bulges are correspondingly smaller.
What about the planets? Venus exerts the greatest gravitational pull on the Earth of all the planets but, at just 0.0054% of the effect of the moon, makes no real impression. Despite being the largest planet, Jupiter's greater distance means that its influence is 10 times smaller than Venus. So the moon and sun are the only celestial bodies that have any significant gravitational impact on the Earth.
More tidal bulges
The orbital motions of the moon and Earth cause the directions to the moon and sun to change over the course of a month and a year respectively. The tilt of the Earth's axis also causes the directions of the moon and sun above and below the Earth's equatorial plane to vary over half of their associated orbital periods. Furthermore, because their orbits are elliptical, the distances to the moon and sun also vary throughout their respective month- and year-long cycles.
The gravitational effect of each of these astronomical cycles can be defined and it is useful to imagine every gravitational variation creating its own unique tidal bulge.
The period of each bulge is identical to that of the gravitational force that created it. These periods therefore, by definition, do not vary and are the same everywhere on Earth. The size, or amplitude, of these bulges is small - the largest having a height of just a few tenths of a metre.
These tidal bulges are known as constituent tides.
Tidal bulges on the move
Since gravitational force acts in a direct line it follows that as the moon and sun move relative to Earth, so each of the direction-related tidal bulges must turn to maintain alignment with the appropriate body.
While the Earth rotates on its axis the moon moves in the same direction around its orbit, turning the lunar tidal bulge as it goes. The following diagram shows the Earth-moon system as viewed from above the North Pole. Initially an observer at position A sees the tidal bulge pointed in the direction of the moon. Twenty-four hours later the observer returns to point A, but during the intervening day the moon has moved along its orbit and rotated the tidal bulge so the observer must wait until reaching point B before catching up with the lunar bulge again.
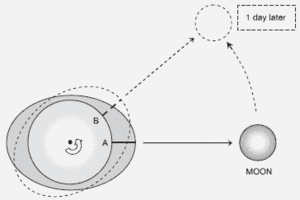
The elapsed time to catch up to the tidal bulge that is directed towards the moon is, on average, 24hr 50min. The time between passes under each tidal bulge (successive high waters) is, therefore, approximately 12hr 25 min. The actual interval can vary by up to 30 minutes.
The tidal bulge caused by and pointing in the direction of the sun is encountered by the rotating Earth after each complete revolution about its axis. Therefore, the interval between successive high waters caused by the solar tide-generating force is 12 hours.
These lunar and solar tide cycles each last half a day and are known as semi-diurnal tides. Since the lunar semi-diurnal tide is dominant over the solar tide, the time of the tide generally advances by nearly one hour each day in accordance with the motion of the moon.
The bulges revolve around the Earth once every 27.32 days in the case of the lunar tidal bulge and 365.24 days for the sun's tidal bulge.
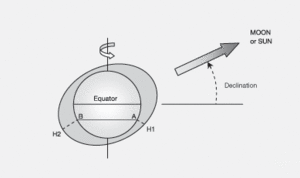
Because the Earth is titled on its axis by approximately 23°, the moon's orbital plane is inclined to the equator by the same angle. During each orbit the declination of the moon changes from 23° on one side of the plane of the Earth's equator to the other over a period of 13.66 days. The lunar tidal bulge follows this motion so we have the situation shown in the following diagram (note - for convenience the Earth's axis is shown in the upright position).
The observer at point A is under a tidal bulge and sees a high tide with height H1. Twelve hours later the observer is at point B and, once again, sees another high tide, this one has height H2. Now H2 is greater that H1 because the declination of the moon has rotated the tidal bulge. This difference between the heights of successive high tides is known as the diurnal inequality. As the moon approaches the plane of the equator, H1 and H2 become equal and the diurnal inequality disappears.
The tidal bulge due to the sun is similarly affected by the Earth's tilt except that the solar cycle lasts 6 months.
The moon's declination also has a secondary oscillation that is a little more than 5°. This additional motion changes very slowly and takes 18.61 years before being repeated.
We have now considered the motion of a number of direction-dependent tidal bulges.
Tidal bulges changing size
As the orbits of the moon about the Earth and the Earth around the sun are not circular, the distance to each of these bodies varies. Since the strength of gravitational attraction is determined, in part, by the distance between the objects, as the distances change so too does the strength of the tide-raising forces.
The lunar distance changes during a period of 27.56 days, which is very close to, but not exactly the same as, the moon's orbital period. As a further complication, the point of the lunar orbit that is closest to the Earth does not stay in the same place. Instead, it moves slowly around the moon's orbit, a journey that takes 8.85 years.
The distance related solar tide-generating force changes over a period of 365.26 days. As is the case with the moon and the Earth, the point where the Earth is closest to the sun also moves around the Earth's orbit, but this is very slow as it takes 20,930 years to complete the circuit.
Combining tidal bulges
Let us now consider what happens when two tidal bulges interact with one another. Since the period of each bulge is different, the rate of change that takes place as they interact will depend on the difference between their periods. When the peaks of both bulges are aligned the height of the resulting bulge will equal the sum of the heights of the individual bulges. When the peak of one bulge is aligned with the trough of the other, then the heights will tend to cancel one another. Bulges with similar amplitudes will have a far greater effect on one another compared to the effect that a small bulge will have on a larger one. The two bulges will create a new tidal bulge whose size (amplitude) and period are predetermined by the original bulges.
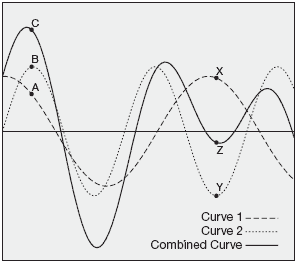
The diagram below illustrates how two simple curves merge together to form a new more complex curve.
The height of curve 1 at point A combines with the height of curve 2 at point B to produce a greater height at point C on the combined curve.
The height of curve 1 at point X is reduced by the negative height of curve 2 at point Y to produce a small negative height on the combined curve at Z.
Spring/neap cycle: This is the most widely known phenomenon resulting from the combination of two tidal bulges. Twice each month the Earth, moon and sun more or less line up and we have what are known as the new and full phases of the moon. At these times the tide generating forces of the moon and sun are aligned. As a result, the major lunar and solar tidal bulges combine together to create spring tide conditions when the difference between the heights of high and low water is greater than average.
The moon continues on its way around its orbit and a week later it is at a right angle to the direction of the sun and the tidal bulges have a cancelling effect on each other. At these times (around first and last quarter moon) the high water is not so high and the low water is not so low - these are known as neap tides.
The tide, as it is observed, is the end result of the combination of many tidal bulges (constituent tides) each of which is linked to a particular set of Earth-moon-sun circumstances.
Fiction versus fact
The foregoing explanation describes what is known as the equilibrium tidal theory that is based on a fictitious water-covered Earth where the tidal bulges remain pointed directly towards and away from the moon and sun. This simplified theory ignores the effects that the continents, varying water depth, rotation and inertia have on the propagation of the tidal bulges.
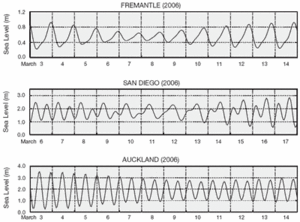
Many of the tidal phenomena observed around the world are not explained by the equilibrium theory. For example, the semidiurnal tide is not universal - some locations experience just one tide a day (a diurnal tidal regime), others have a mixed regime where times of semi-diurnal tides alternate with periods of diurnal tides. Examples of diurnal tides (Fremantle) and mixed tides (San Diego) are shown below together with Auckland's semi-diurnal regime for comparison.
Tide ranges many times greater than predicted by the equilibrium theory are not exceptional. In some places the range can exceed 10 metres (for example Broome and Derby, Western Australia and the Bay of Fundy, Canada) while elsewhere such as the Mediterranean, Baltic and Caribbean Seas the tide is almost non-existent. The spring/neap cycle is a dominant feature of the tides in some regions; in others this twice-monthly rhythm is either not so apparent or reduced to a single cycle per month.
Despite these shortcomings, the equilibrium theory does provide some understanding of the nature of the connection between the tides and the moon and sun and it forms the basis of the concepts underlying the constituent tides.
The effect of the real world on the tidal bulges
The arrangement of the continents has created the world's three major water basins - the Pacific, Atlantic and Indian Oceans. On the scale of these oceans, the passages of connecting water are relatively small and so, broadly speaking, the movement of the tide is constrained within individual ocean basins. The tidal bulge, as developed by the equilibrium theory, must now be considered as a very long wave trapped within each basin.
Each body of water has a natural period of oscillation which will influence its response to the tide raising forces. In general, the oscillation period for the Pacific Ocean is 25 hours and so many of the tides in the Pacific are diurnal. In the Atlantic Ocean the natural oscillation period is about 12½ hours so the tides in the Atlantic are predominantly semi-diurnal. There are, of course, many places where the tidal regime is governed by the local configuration of the land and water depth rather than large-scale oceanic forces.
If you take a container of water and move it from side to side, the water level at each end will rise and fall, while across the centre of the container the water level will not change. This over-simplified model describes the nature of the tides in enclosed waters such as the Persian Gulf, Red Sea and Mediterranean Sea.
Now move the container about in a circular fashion. The water level will rise and fall around the edge of the container and the location where the water level does not change is reduced to a point in the centre of the container. This pattern of wave behaviour is similar to what happens within the ocean basins as the Earth rotates. In the northern hemisphere the long tide wave travels in an anti-clockwise direction, while the wave in the southern hemisphere goes clockwise. The centre of these Coriolis rotations is known as an amphidrome, a point where there is no tide.
The speed at which a wave travels is controlled by water depth. Those parts of the wave passing over continental shelf regions and shallower coastal areas will slow down and this, in turn, means that the wave front no longer remains straight. The slowing of the wave also causes the wave height to increase. According to the equilibrium tide theory, the height of the tide wave is less than half a metre. Slowing the wave in shallow water produces observed tide heights that are many times this value.